Andreas Winter – Universitat Autònoma de Barcelona (UAB)
A reference frame is commonly understood as a convention to give objective meaning to relative information such as time and distance. In modern physics, however, it is a concrete, rigid external structure to allow for the unambigous definition of such quantities as angles or lengths in terms of measurements. Thus, Einstein imagined a universe full of clocks and rods, but the role of a frame is not reducible to merely giving operational sense to observables through measurement. Indeed, in quantum mechanics, not all quantities can be measured simultaneously, yet frames exist for all sets of quantum numbers, regardless of whether they are jointly measureable or not (in mathematical terms: do or do not commute). In [1] we gave a simple and universal construction of frames for arbitrary quantum numbers. Previously, frames were known based on abstract representationtheoretic principles. Their crucial feature is that they enable arbitrary transformations on a system (including measurements), even ones that do not conserve the observables of interest, offsetting each change by an opposite change in the reference frame. The latter thus necessarily acts as a battery, a very useful point of view in thermodynamics.
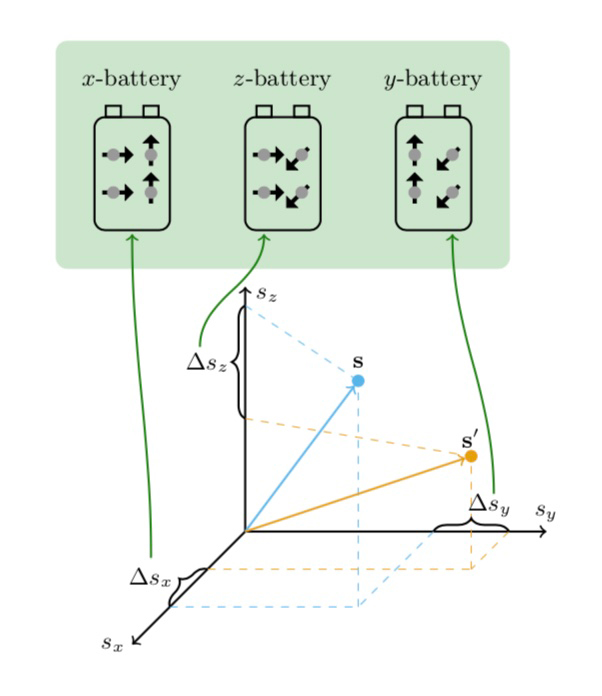
It turns out that there is much freedom in constructing frames, and that this freedom can be exploited to impose additional desirable features. In thermodynamics the question had arisen whether there are frames with physically distinct parts serving as batteries for the different quantum numbers in question.
We show that this is indeed the case in several interesting cases [2]. E.g. for angular momentum (Fig. 1), one needs a 3-dimensional coordinate system; a frame could be three large spins pointing in (roughly) orthogonal directions. Alternatively, each of the three directions can be given indirectly via the right-hand rule based on two large spins (Fig. 2). Whereas the first offsets any change of the principal spin components by an opposite change of declocalized internal degrees of freedom, the latter frame has three physically distinct parts for each of the principal spin components.